| Ch 2. Particle Force and Acceleration | Multimedia Engineering Dynamics | ||||||
|
Rect. Coord. |
Normal/Tang. Coord. |
Polar Coord. |
Orbital Mechanics | Computational Mechanics | |||
| Normal/Tangential Coordinates | Case Intro | Theory | Case Solution | Example |
| Chapter |
| - Particle - |
| 1. General Motion |
| 2. Force & Accel. |
| 3. Energy |
| 4. Momentum |
| - Rigid Body - |
| 5. General Motion |
| 6. Force & Accel. |
| 7. Energy |
| 8. Momentum |
| 9. 3-D Motion |
| 10. Vibrations |
| Appendix |
| Basic Math |
| Units |
| Basic Dynamics Eqs |
| Sections |
| eBooks |
| Dynamics |
| Fluids |
| Math |
| Mechanics |
| Statics |
| Thermodynamics |
| ©Kurt Gramoll |
|
|
||
| Introduction |
||
|
|
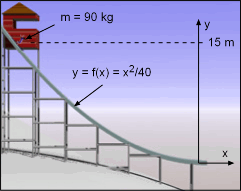
Just prior to the Winter Olympics, the Olympic committee must erect a 15-meter ski jump. If the jump is not sturdy enough, the force of the skier could cause the jump to collapse. What is known:
|
|
 Problem Graphic |
Question |
|
|
At the end of the jump, what is the normal force exerted on the skier by the jump? What is the total acceleration of the skier at the end of the jump? |
||
| Approach |
||
|
||