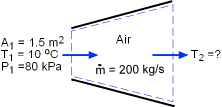
Air temperature at the exit of the wind tunnel needs to be determined to see if it is safe to exhaust the air to ambient.

Assumptions:

• Air is an ideal gas
• Heat transfer is negligible
• No change in potential energy
• No work interactions

Consider the Diffuser as a
Control Volume

(1) Determine the velocity at the inlet of the diffuser

To determine the velocity at the inlet, the specific volume of the air needs to be first determined. Since the air in the tunnel is an ideal gas, it obeys the ideal-gas equation of state.

      Pv = RT

where
       R = 287 J/(kg-K) for air
       v = specific volume of the air

The specific volume can be determined at the inlet conditions:

      v₁ = RT₁/P₁ = 287(273+10)/80,000 = 1.015 m³/kg

The velocity can be calculated using the following equation:

      v₁ = v₁/A₁ =200(1.015)/1.5 =135.3 m/s

(2) Determine the temperature at the exit of the diffuser

Under the stated assumptions and observations, the energy balance for the steady-flow through the diffuser can be expressed as

      (h₂ - h₁) + ( v₂² - v₁²)/2 = 0

      h₂ = h₁ - ( v₂² - v₁²)/2

The enthalpy of air at the diffuser inlet can be determined from the air table to be

      h₁ = h_{@283 k} = 283.14 kJ/kg

Assume the velocity at the exit is 0, then the enthalpy reaches the maximum value.

      h₂ = 283.14 - ( 0² - 135.3²)/2/1,000 = 292.3 kJ/kg

From the air table, the temperature corresponding to this enthalpy value is

      T₂ = 292 K = 19^oC < 35 ^oC

which shows that the air is safe to exhaust to the outside environment.