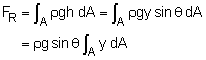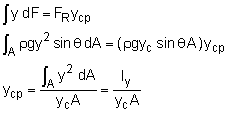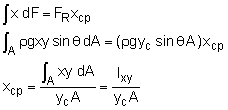Hydrostatic Force on an
Inclined Arbitrary Plane Surface

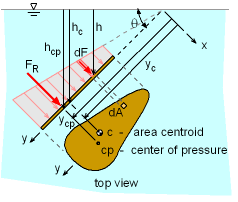
Consider an inclined plane submerged in a static fluid as shown in the figure. The resultant force F_R is acting perpendicular to the plane since no shear force is present when the fluid is at rest. F_R has a line of action that passes through the point (x_cp , y_cp), which is called the center of pressure. Note that the pressure acting perpendicular to the plane surface is also referred to as the normal stress.

Now take a small differential element dA at a depth of h. The differential force dF acting on dA is given by

     dF = ρgh dA

The magnitude of the resultant force can be obtained by integrating the differential force over the whole area

The integral represents the first moment of the area about the x axis, which is equal to

where y_c is the y coordinate of the centroid of the plane surface.

From trigonometry,

     h_c = y_c sinθ

where h_c is the vertical distance from the fluid surface to the centroid of the plane surface. The resultant force is simplified to

The center of pressure, x_cp and y_cp can be obtained by summing moments about the y and x axis, respectively. First, by equating the sum of moments of all pressure forces about the x axis to the moment of the resultant force:

where is the second moment of the area or the area moment of inertia (I_x) about the x axis. According to the parallel axis theorem, the moment of inertia can also be written as

     I_x = I_x' + Ay_c²

where I_x' is the second moment of the area with respect to the centroidal axis, which is parallel to the x axis.

Hence, the center of pressure coordinate y_cp is given by

Similarly, x_cp can be obtained by equating the sum of moments of all pressure forces about the y axis to the moment of the resultant force

where is the product of inertia (I_xy) of the area about the x and y axes. Once again according to the parallel axis theorem, it can also be written as

     I_xy = I_xy' + Ax_cy_c

where I_xy' is the product of inertia of the area with respect to the centroidal axes.

Hence, the coordinate x_cp is given by

The values for the second moment of inertia and product of inertia with respect to the centroidal axes for various common geometries are given in the appendix. From the formulations of x_cp and y_cp, it is noted that the center of pressure is always lower in the liquid than the centroid of the plane area.

Hydrostatic Pressure Force Exerted
on a Vertical Plane Surface



Pressure Prism

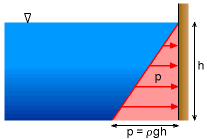
An alternate approach of determining the hydrostatic force is by means of a pressure prism. Consider a vertical plane submerged in a static fluid, as shown in the figure. The pressure increases linearly with the depth. One can then easily construct a corresponding three-dimensional diagram of the pressure distribution, and such a volume is called a pressure prism. The resultant force is the total volume of the pressure prism, that is

     F_R = Volume = 1/2 (ρgh) (bh)

The resultant force passes through the centroid of the pressure prism. For this particular example, the centroid of a triangular element is located at a distance of h/3 from its base and lies in the vertical symmetry axis.

As illustrated, this method is particularly convenient when the shape of the pressure prism is a common geometry, in which the volume and centroid can be readily obtained.